William Rowan Hamilton— Ireland’s liberator of algebra
Published in 18th-19th Century Social Perspectives, Features, Issue 5 (September/October 2015), Volume 23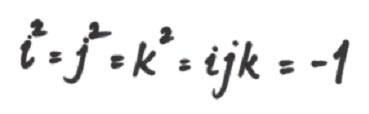
The formula for quaternions scratched by William Rowan Hamilton on Broombridge, Cabra, 16 October 1843.
‘ONE SMALL SCRATCH FOR A MAN, ONE GIANT LEAP FOR MATHEMATICS’
On a bright Monday morning, on 16 October 1843, the world of mathematics was changed forever. William Rowan Hamilton was walking along the banks of the Royal Canal in Dublin with his wife, Helen. As he passed Broombridge in Cabra, he had a flash of inspiration.
Hamilton described the ‘eureka’ moment in a letter to his son some years later:
‘Although your mother talked with me now and then, yet an undercurrent of thought was going on in my mind, which gave at last a result, whereof it is not too much to say that I felt at once an importance. An electric current seemed to close; and a spark flashed forth, the herald (as I foresaw, immediately) of many long years to come of definitely directed thought and work . . . Nor could I resist the impulse—unphilosophical as it may have been—to cut with a knife on a stone of Brougham Bridge as we passed it, the fundamental formula . . .’
Thus Hamilton’s mind gave birth to a strange new system of four-dimensional numbers called ‘quaternions’. In a nineteenth-century act of minor vandalism, Hamilton scratched his mathematical creation on the bridge.
Liberator of algebra
The quaternions are Hamilton’s most celebrated contribution to mathematics. Two-dimensional numbers had played a significant role in two-dimensional geometry and in solving practical problems in two dimensions. Hamilton had been trying to extend his theory of two-dimensional numbers to a theory of three-dimensional numbers (also called triplets). He hoped that these triplets would provide a natural mathematical structure for describing our three-dimensional world. He had difficulty finding a suitable theory of triplets (we now know why—it’s impossible!). Then, on 16 October 1843, his mind gave birth to quaternions as he walked along the banks of the Royal Canal. In this moment of revelation he realised that if he worked with number quadruples and an unusual multiplication operation he would get everything he wanted. He named his new system of numbers ‘quaternions’ because each number quad-ruple had four components.
Hamilton had created a completely new structure in mathematics. The mathematical world was stunned by his audacity in creating a system of numbers that did not satisfy the usual commutative rule for multiplication. (This is the rule in arithmetic which says that it does not matter in which order you multiply two ordinary numbers, e.g. two times three equals three times two. The quaternions did not satisfy this rule.) This did not bother Hamilton because this is what usually happens in nature. For example, consider an empty swimming pool and the two operations of diving into the pool head first and turning the water on. The order in which the operations take place is important!
Hamilton has been called the ‘liberator of algebra’ because his quaternions smashed the previously accepted notion that any useful algebraic number system should satisfy the rules of ordinary numbers in arithmetic. His quaternions opened up a new landscape where mathematicians could feel free to conceive new number systems that were not shackled by the rules of ordinary numbers in arithmetic. One could say that modern algebra was born on the banks of the Royal Canal in Dublin. I suppose one could also say that it was ‘one small scratch for a man, one giant leap for mathematics’! Hamilton’s creation of quaternions is commemorated by a plaque which was unveiled in 1958 by the taoiseach, Éamon de Valera, who was a mathematician himself and a fan of Hamilton. De Valera paid homage to Hamilton by scratching the quaternion formulas on his cell wall when he was in Kilmainham Jail in 1924.
Use of the word ‘liberator’ illustrates the fact that freedom plays a significant role in mathematics. The notion of freedom in mathematics can surprise some people, but the mathematician Cantor once said that freedom is the essence of mathematics. In fact, one is free to conceive of any new ideas one wants in mathematics (just as Hamilton was free to conceive of the quaternions, even though they broke with convention at the time). These new ideas may or may not lead to something interesting or useful. Historically (and probably also in the future) the major breakthroughs in mathematics have typically happened because the great mathematicians were free to conceive of any new ideas they wanted, even if their wild thoughts broke with conventions and seemed bizarre to other mathematicians and the general public.
Applications of Hamilton’s mathematics
Hamilton’s mathematics has been, and still is, crucial for many important applications to science, engin-eering, computer animation, computer games, special effects in movies, space navigation and many other areas.
For example:
(a) Lara Croft in Tomb Raider was created using quaternions.
(b) Quaternions now play an important role in special effects in movies. For example, an Irish company called Havok used quaternions in the creation of the acclaimed new special effects in The Matrix Reloaded, and also in Poseidon, which was nominated for an Oscar for its visual effects in 2007. Havok won an Emmy award in the US in 2008 for pioneering new levels of realism and interactivity in movies and games. The dramatic visual effects in the James Bond movie Quantum of Solace were also created by Havok.
(c) Space navigation uses quaternions. For example, quaternions were fundamental in the successful operation of Apollo 11, which landed the first man on the moon in 1969. Quaternions were also crucial in the landing of Curiosity on Mars in 2012.
(d) Quaternions played an important role in Maxwell’s mathematical theory and prediction of electromagnetic waves in 1864. Maxwell’s theory ultimately led to the detection of radio waves by Hertz. Thus the inventions of radio, television, radar, X-rays and many other significant products of our time are directly related to Hamilton’s mathematics. Maxwell’s work illustrates the magical power of mathematics because his mathematics made the invisible visible, since radio waves are invisible to our five senses.
(e) Hamilton’s fundamental theory of dynamics in 1834 was indispensable for the creation, in the early 1900s, of Quantum Mechanics, which is how we now understand the physical world at the microscopic level. Moreover, his famous Hamiltonian function is fundamental to many aspects of physics.
(f) Vector analysis, which is indispensable in physics, is an offspring of quaternions.
Inspiration for artists and more
Hamilton’s story is a great example of something from the history of mathematics that continues to inspire people (many of whom are outside mathematics). There is a wide diversity of public responses to Hamilton’s story, including sculptures (busts, bog oak and sand), paintings, poems, stamps, plaques, graffiti art, a song, a statue, a coin, a walk, a video installation in an art gallery, TV documentaries, radio shows, newspaper articles, housing areas, institutes, T-shirts, a building, a moon crater, a golf hole, a banner, a bridge, and references in James Joyce’s Finnegans Wake (‘… wondering was it hebrew set to himmeltones or the quicksilversong of qwaternions; his troubles may be over but his doubles have still to come’), Flann O’Brien’s work and a play by Sebastian Barry.
Fiacre Ó Cairbre is a Senior Lecturer in Mathematics at Maynooth University.
Background
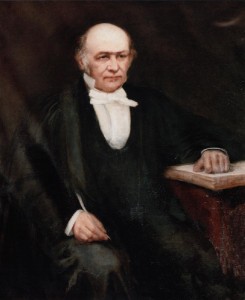
William Rowan Hamilton. (RIA)
William Rowan Hamilton was born in Dominick Street, Dublin, on 4 August 1805 and spent his early youth on the banks of the Boyne in Trim, Co. Meath, across from the spectacular ruins of Trim Castle. He then lived in Dunsink Observatory in Dublin for the rest of his life. Like most great mathematicians, his motivation was the quest for beauty. Typically, the beauty in mathematics lies in the beauty of ideas, because mathematics essentially comprises an abundance of ideas. I consider beauty to be the most important feature in mathematics. Hamilton was also a poet, and poetry played a prominent role in his life. He won the Chancellor’s poetry prize twice in Trinity College, Dublin, and his literary work was published in journals and magazines. Hamilton regarded mathematics ‘as an aesthetic creation, akin to poetry, with its own mysteries and moments of profound revelation’.
The annual Hamilton walk

Professor A.G. O’Farrell speaking at Broombridge during the 2005 walk.
The annual Hamilton walk, initiated by Professor Anthony G. O’Farrell in 1990, takes place on 16 October to commemorate Hamilton’s famous creation. It retraces his steps from Dunsink Observatory to Broombridge and takes about 45 minutes. I organise the walk, which typically attracts over 200 people from diverse backgrounds, including staff and students from third level, staff and students from second level, and many from the general public. The large number of participants from the general public indicates that there is substantial interest in Hamilton and the walk. I also receive many calls from the media and other bodies every year expressing an interest in doing a piece on Hamilton and the walk. Consequently, Hamilton’s story and the walk have appeared many times on a variety of television and radio programmes and in lots of news-paper articles. Cabra Community Council make the event into a very festive affair, with a large banner draped across the bridge and stalls along the canal. Fields Medallists Timothy Gowers and Efim Zelmanov, and Nobel Prize-winners in physics Murray Gell-Mann, Steven Weinberg and Frank Wilczek have participated in the walk. Broombridge has become a world-famous site in the history of mathematics and science because of Hamilton’s creation of quaternions. The word ‘Broomsday’ is now sometimes used in mathematical and scientific circles to indicate 16 October, and plays the same role as Bloomsday does for literary groups.
Contact Fiacre Ó Cairbre, fiacre.ocairbre@nuim.ie, +353 (0)1 7083763.
Further reading
F. Ó Cairbre, ‘William Rowan Hamilton (1805–1865): Ireland’s greatest mathematician’, Ríocht na Midhe (2000).
F. Ó Cairbre, ‘Twenty years of the Hamilton walk’, Irish Mathematical Society Bulletin (2010).
F. Ó Cairbre, ‘Mathematics education and the public’s response to the Hamilton story’, Irish Mathematical Society Bulletin (2014).
















